PC2-3
Self-consistent Study of Topological Superconductivity in Two-dimensional Quasicrystals
*Masahiro Hori1,2, Rasoul Ghadimi3, Takanori Sugimoto1, Takami Tohyama1, K Tanaka2
- Department of Applied Physics, Tokyo University of Science, Tokyo 125-8585, Japan1
- Department of Physics and Engineering Physics, and Centre for Quantum Topology and Its Applications (quanTA), University of Saskatchewan, 116 Science Place, Saskatoon, Saskatchewan, Canada S7N 5E21
- Center for Correlated Electron Systems, Institute for Basic Science, Seoul National University, 1, Gwanak-ro, Gwanak-gu, Seoul, Korea1
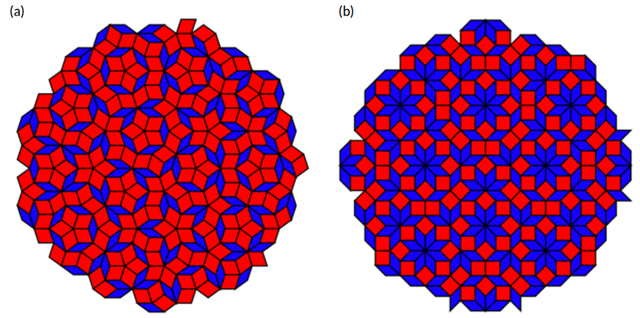
Motivated by the recent discovery of superconductivity in a quasicrystal [1], we investigate the occurrence of topological superconductivity (TSC) in two-dimensional (2D) quasicrystals. Quasicrystals are characterized by long-range order without periodicity. The most fundamental examples are Penrose (Fig. 1(a)) and Ammann-Beenker tilings (Fig. 1(b)). The Penrose tiling is composed of two rhombuses, colored by red and blue in Fig. 1(a). Similarly, the Ammann-Beenker tiling consists of a square and a rhombus, colored by red and blue, respectively, in Fig. 1(b). Quasicrystals are inherently fractal, characterized by self-similarity. Theoretically, so far TSC has been studied mainly in periodic crystals such as square lattice systems. Although there is a previous study about TSC in two-dimensional quasicrystals, the uniform order parameter was forcely assumed [2]. Therefore, it is not trivial whether a stable TSC phase can exist in quasicrystals, despite their aperiodic and fractal structure.
We solve the Bogoliubov-de Gennes equations on the tight-binding model for 2D TSC of Sato, Takahashi, and Fujimoto [3] generalized for quasicrystals self-consistently [4]. This model [3] describes 2D TSC with broken time-reversal symmetry as experimentally realized [5]. According to the symmetry classification by Schnyder et al. [6], this model is in class D, which has an integer classification given by the TKNN number.
By solving for the superconducting order parameter self-consistently, we examine the stability of TSC in quasicrystals. Furthermore, in terms of our self-consistent solutions that properly reflect the local environment of each lattice site (vertex) in a quasicrystal and hence its self-similarity, we explore possible fractal structure in the distribution of order parameters.
References:
[1] K. Kamiya et al., Nat. Comm. 9, 154 (2018).
[2] R. Ghadimi, T. Sugimoto, K. Tanaka, T. Tohyama, arXiv:2006.06952.
[3] M. Sato, Y. Takahashi, S. Fujimoto, Phys. Rev. B 82, 134521 (2010).
[4] S. L. Goertzen, K. Tanaka, Y. Nagai, Phys. Rev. B 95, 064509 (2017).
[5] G. C. Ménard et al., Nat. Comm. 8, 2040 (2017).
[6] A. P. Schnyder et al., Phys. Rev. B 78, 195125 (2008).
Figure Caption:
Fig. 1. Two of the most fundamental examples of quasicrystals: (a) Penrose tiling, (b) Ammann-Beenker tiling
Keywords: Topological superconductivity, Quasicrystals, Penrose tiling, Ammann-Beenker tiling