For applications of superconductors it is important to control vortices. However, dynamics of vortices in a dirty super conductor under an external current is still not clean. For example, Nishida et al. showed vortices move as bundles separated by glide plane of dislocation[1]. In order to clarify vortex dynamics ,we have developed “Molecular + Field Dynamics method.”

In a usual molecular dynamics (MD) method, an equation of motion for the i-th vortex is given by
ηdr_i/dt=f^d_i+f^p_i+f^v_i+f^f_i
where η is viscosity coefficient, r_i is position of the vortex, f^d_i is a driving force by an external current, f^p_i is a pinning force due to an impurity potential, f^v_i is a repulsive force from other vortices, and f^f_i is a force due to thermal fluctuations.

In this method, we can only consider a few thousands vortices because of computation time of repulsive interactions between all points of vortices.

In order to treat more than ten thousands vortices, we consider a current field that consists of an external current and current around vortices.

The driving force from the external current and repulsive force from other vortices can be calculated locally as a current force fCi. Then the equation of motion becomes
ηdr_i/dt=f^C_i+f^p_i+f^f_i
The current field is obtained using the finite element method.In the simulations, a square superconductor was assumed, and the length of a piece was set to 5λ₀ where λ₀ is magnetic field penetration length.

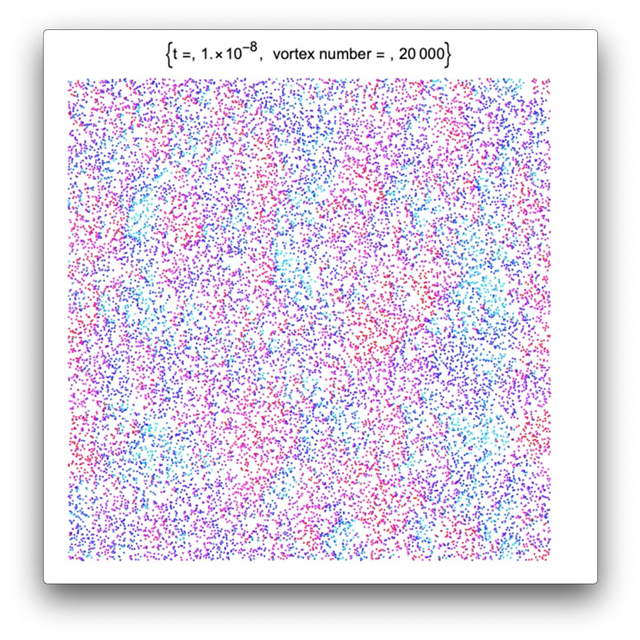
Figure1 shows a vortex distribution from a simulation of 20000 vortices. Colors of vortices show direction of velocities of vortices.Using this method, we will show collective motions of vortices.

[1]N. Nishida, K. Hirata, H. Takeda and H. Takeya, Physica C 470(2010)S795-S796

Keywords: Molecular dynamics method, Vortex, Finite Element method